Two new pieces of research into 3D structures could have novel applications in 3D printing.
Massachusetts Institute of Technology (MIT) researchers Erik Demaine and Tomohiro Tachi have developed an algorithm to fulfil the infinite probabilities of folding a 2D sheet of paper into a 3D shape.
The algorithm is the result of 20 years of work. By applying this theory to sheets of other materials, e.g. PLA, ABS and Nylon, the algorithm may have potential to enhance the possibilities of 3D printed objects, including self-assembling 4D printing.
Also in this article looking at the design of 3D structures we report on new research from the Institute of Science and Technology Austria (IST Austria) into self-folding 3D structures. The work focuses on overcoming challenges to the surface smoothness of self-actuating objects.
The ultimate origami algorithm
In computer aided design (CAD) all 3D objects are made out of a mesh of multiple polygons. At its most basic level, Demaine and Tachi’s algorithm takes these polygons and applies them to a 2D sheet of material. In addition, it accounts for the seams between polygons when folded, ensuring that they are hidden and don’t obstruct the geometry of the overall shape.

As a comprehensive study, the algorithm is mathematically complex, indicative of the 20 years of research it has taken to get to this stage of the process. Speaking to Rich Haridy at New Atlas Robert Lang, an origami expert and physicist, responds to the research, saying,
Along the way, there have been several nice demonstrations of pieces of the puzzle: an algorithm to fold any shape, but not very efficiently; an algorithm to efficiently fold particular families of tree-like shapes, but not surfaces; an algorithm to fold trees and surfaces, but not every shape. This one covers it all! The algorithm is surprisingly complex, but that arises because it is comprehensive. It truly covers every possibility. And it is not just an abstract proof; it is readily computationally implementable.
The principle in practice
As assistant professor in Graphic and Computer Sciences at the University of Tokyo, Tachi plans to incorporate the algorithm into Origamizer 3D design software. Originally released in 2008, Origamizer facilitates the creation of complex paper shapes from 3D models.
Demo of Origamizer software used to generate the folds needed to create a 3D donut shape. Clip via Tomohiro Tachi on YouTube
In principle, this technology could also enable 3D designers to generate a flat “map” of any 3D shape. This map can then be 3D printed in a fraction of the time, using less material than your typical 3D print, and folded into its final form after printing.
Furthermore, the properties of the 3D printed material could be tuned to enable the flat “map” to self-actuate into a pre-programmed form.
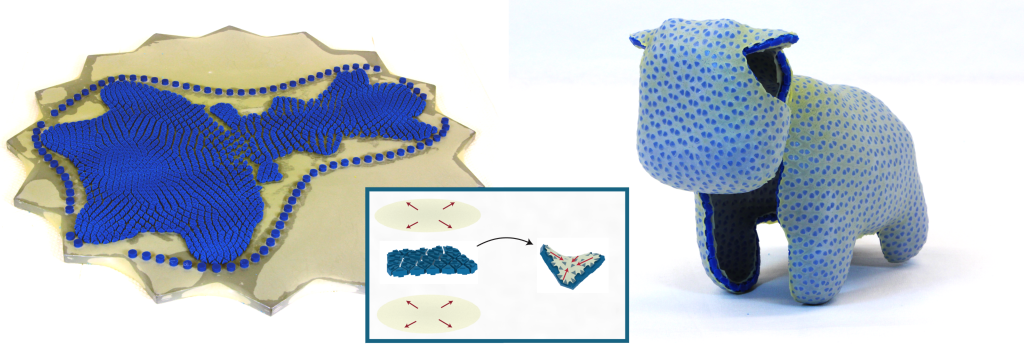
IST Austria’s smooth surface of CurveUps
The term 4D printing was first coined in 2013 by Skylar Tibbits, director and founder of the Self-Assembly Lab at MIT.
It refers to the use of 3D printing to create a flat net shape that, when certain conditions such as heat or moisture are applied, transforms into a 3D object. 4D printing has been applied to a number of structures created at MIT’s Self-Assembly Lab and elsewhere around the world. For example, ETH Zurich’s “deployable and active” truss research and in bucky ball assembly by researchers in Singapore.
Having proved its viability, a further challenge to 4D printing is in the ability to create self-actuating structures that also have a smooth surface, independent of the straight lines and angular shapes used in their composition. With “CurveUps” researchers at IST Austria have realized this potential, creating 2D maps that can self actuate into a number of rounded forms.

The full study supporting the CurveUp technology is available to read open-access via the Visual Computing department at IST Austria here. It is co-authored by Ruslan Guseinov, Eder Miguel and Bernd Bickel. A video explaining the process is also available via Ruslan Guseinov on YouTube.
Stay up-to-date with the latest 3D printing news and research by subscribing to our newsletter and following us on twitter and Facebook.
Featured image: Erik Demaine’s analysis of the straight-skeleton origami method. Image via erikdemaine.org



